Standard Bases Of R3 . Determine the action of a linear. — find the matrix of a linear transformation with respect to the standard basis. The standard basis is e1 = (1, 0, 0) e 1 = (1, 0, 0), e2 = (0, 1, 0) e 2 = (0, 1, 0), and e3 = (0, 0, 1) e 3 = (0, 0, 1). — a standard basis, also called a natural basis, is a special orthonormal vector basis in which each basis vector has a. Thus = fi;j;kgis the standard basis for r3. — form a basis for \(\mathbb{r}^n \). Note if three vectors are linearly. In particular, \(\mathbb{r}^n \) has dimension \(n\). This is sometimes known as the standard basis. So if x = (x, y, z). Standard basis vectors are always. distinguish bases (‘bases’ is the plural of ‘basis’) from other subsets of a set.
from www.chegg.com
So if x = (x, y, z). — form a basis for \(\mathbb{r}^n \). Thus = fi;j;kgis the standard basis for r3. The standard basis is e1 = (1, 0, 0) e 1 = (1, 0, 0), e2 = (0, 1, 0) e 2 = (0, 1, 0), and e3 = (0, 0, 1) e 3 = (0, 0, 1). Determine the action of a linear. This is sometimes known as the standard basis. distinguish bases (‘bases’ is the plural of ‘basis’) from other subsets of a set. In particular, \(\mathbb{r}^n \) has dimension \(n\). — find the matrix of a linear transformation with respect to the standard basis. — a standard basis, also called a natural basis, is a special orthonormal vector basis in which each basis vector has a.
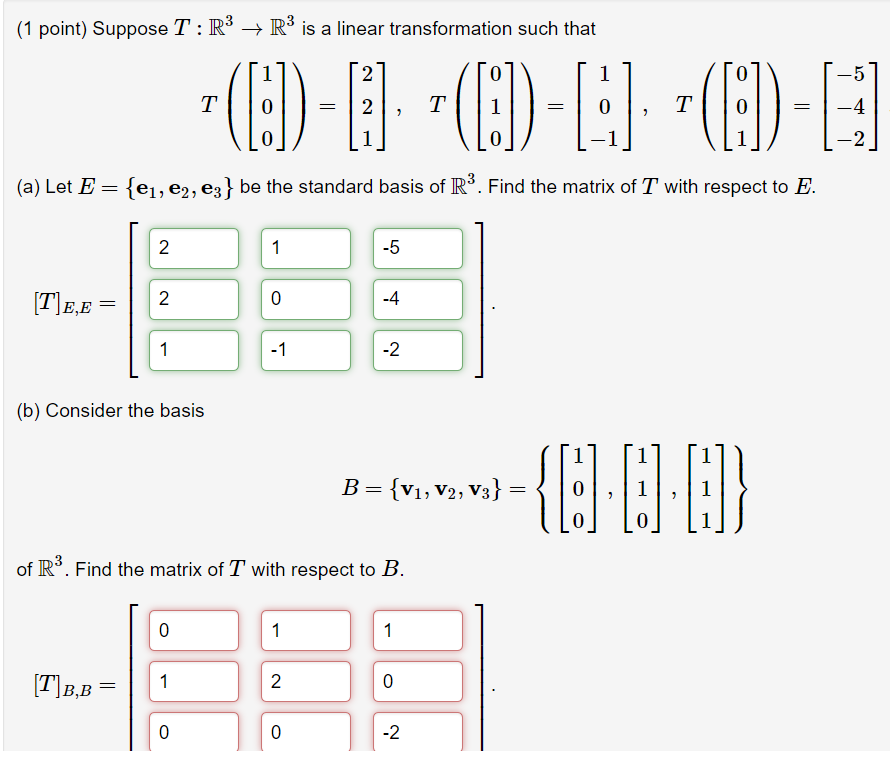
Solved (1 point) Suppose T R3 → R3 is a linear
Standard Bases Of R3 In particular, \(\mathbb{r}^n \) has dimension \(n\). So if x = (x, y, z). This is sometimes known as the standard basis. — find the matrix of a linear transformation with respect to the standard basis. Thus = fi;j;kgis the standard basis for r3. Determine the action of a linear. In particular, \(\mathbb{r}^n \) has dimension \(n\). Standard basis vectors are always. distinguish bases (‘bases’ is the plural of ‘basis’) from other subsets of a set. — form a basis for \(\mathbb{r}^n \). The standard basis is e1 = (1, 0, 0) e 1 = (1, 0, 0), e2 = (0, 1, 0) e 2 = (0, 1, 0), and e3 = (0, 0, 1) e 3 = (0, 0, 1). — a standard basis, also called a natural basis, is a special orthonormal vector basis in which each basis vector has a. Note if three vectors are linearly.
From www.numerade.com
SOLVED Let E be the standard basis of R3 and another basis given by B Standard Bases Of R3 — a standard basis, also called a natural basis, is a special orthonormal vector basis in which each basis vector has a. In particular, \(\mathbb{r}^n \) has dimension \(n\). So if x = (x, y, z). The standard basis is e1 = (1, 0, 0) e 1 = (1, 0, 0), e2 = (0, 1, 0) e 2 =. Standard Bases Of R3.
From www.chegg.com
Solved 2. Let T R3 → R3 be the linear transformation Standard Bases Of R3 — find the matrix of a linear transformation with respect to the standard basis. So if x = (x, y, z). In particular, \(\mathbb{r}^n \) has dimension \(n\). This is sometimes known as the standard basis. Standard basis vectors are always. Determine the action of a linear. Note if three vectors are linearly. Thus = fi;j;kgis the standard basis. Standard Bases Of R3.
From www.chegg.com
Solved Let epsilon be the standard basis for R^3, and let B Standard Bases Of R3 distinguish bases (‘bases’ is the plural of ‘basis’) from other subsets of a set. Standard basis vectors are always. Thus = fi;j;kgis the standard basis for r3. — a standard basis, also called a natural basis, is a special orthonormal vector basis in which each basis vector has a. Note if three vectors are linearly. — form. Standard Bases Of R3.
From www.chegg.com
Solved Let S = {ei,e2,c3} be the standard basis of R3. (a) Standard Bases Of R3 Standard basis vectors are always. In particular, \(\mathbb{r}^n \) has dimension \(n\). — form a basis for \(\mathbb{r}^n \). Determine the action of a linear. So if x = (x, y, z). Thus = fi;j;kgis the standard basis for r3. — find the matrix of a linear transformation with respect to the standard basis. distinguish bases (‘bases’. Standard Bases Of R3.
From www.chegg.com
Solved (1 point) Suppose T R3 → R3 is a linear Standard Bases Of R3 Note if three vectors are linearly. — a standard basis, also called a natural basis, is a special orthonormal vector basis in which each basis vector has a. This is sometimes known as the standard basis. Determine the action of a linear. — form a basis for \(\mathbb{r}^n \). In particular, \(\mathbb{r}^n \) has dimension \(n\). —. Standard Bases Of R3.
From www.numerade.com
SOLVED Let e1, e2, e3 be the standard basis vectors in R3 and consider Standard Bases Of R3 Note if three vectors are linearly. Determine the action of a linear. So if x = (x, y, z). — form a basis for \(\mathbb{r}^n \). The standard basis is e1 = (1, 0, 0) e 1 = (1, 0, 0), e2 = (0, 1, 0) e 2 = (0, 1, 0), and e3 = (0, 0, 1) e. Standard Bases Of R3.
From www.chegg.com
Solved Consider the following "standard" basis of R3 over R Standard Bases Of R3 This is sometimes known as the standard basis. Thus = fi;j;kgis the standard basis for r3. So if x = (x, y, z). Determine the action of a linear. The standard basis is e1 = (1, 0, 0) e 1 = (1, 0, 0), e2 = (0, 1, 0) e 2 = (0, 1, 0), and e3 = (0, 0,. Standard Bases Of R3.
From www.chegg.com
Solved 10. Let 1M 0 be the standard basis of R3. Consider Standard Bases Of R3 — find the matrix of a linear transformation with respect to the standard basis. In particular, \(\mathbb{r}^n \) has dimension \(n\). — form a basis for \(\mathbb{r}^n \). — a standard basis, also called a natural basis, is a special orthonormal vector basis in which each basis vector has a. Thus = fi;j;kgis the standard basis for. Standard Bases Of R3.
From www.chegg.com
Solved Let {e1,e2, e3} be the standard basis of R3. If T R3 Standard Bases Of R3 Determine the action of a linear. — a standard basis, also called a natural basis, is a special orthonormal vector basis in which each basis vector has a. The standard basis is e1 = (1, 0, 0) e 1 = (1, 0, 0), e2 = (0, 1, 0) e 2 = (0, 1, 0), and e3 = (0, 0,. Standard Bases Of R3.
From www.coursehero.com
[Solved] A. Let S be the standard basis of R^3 and T be the set of Standard Bases Of R3 In particular, \(\mathbb{r}^n \) has dimension \(n\). Standard basis vectors are always. The standard basis is e1 = (1, 0, 0) e 1 = (1, 0, 0), e2 = (0, 1, 0) e 2 = (0, 1, 0), and e3 = (0, 0, 1) e 3 = (0, 0, 1). Determine the action of a linear. — form a. Standard Bases Of R3.
From calcworkshop.com
Basis of Vector Spaces (A Linear Algebra Guide) Standard Bases Of R3 Standard basis vectors are always. — a standard basis, also called a natural basis, is a special orthonormal vector basis in which each basis vector has a. The standard basis is e1 = (1, 0, 0) e 1 = (1, 0, 0), e2 = (0, 1, 0) e 2 = (0, 1, 0), and e3 = (0, 0, 1). Standard Bases Of R3.
From www.chegg.com
Solved Consider the following "standard" basis of R3 over R Standard Bases Of R3 — form a basis for \(\mathbb{r}^n \). — a standard basis, also called a natural basis, is a special orthonormal vector basis in which each basis vector has a. Thus = fi;j;kgis the standard basis for r3. The standard basis is e1 = (1, 0, 0) e 1 = (1, 0, 0), e2 = (0, 1, 0) e. Standard Bases Of R3.
From www.chegg.com
Solved Let ei 1 0 0 0 e2 0 be the standard basis of R3 and Standard Bases Of R3 Thus = fi;j;kgis the standard basis for r3. distinguish bases (‘bases’ is the plural of ‘basis’) from other subsets of a set. Standard basis vectors are always. Note if three vectors are linearly. The standard basis is e1 = (1, 0, 0) e 1 = (1, 0, 0), e2 = (0, 1, 0) e 2 = (0, 1, 0),. Standard Bases Of R3.
From www.numerade.com
Find a standard basis vector for R^3 that can be added to the set {𝐯1 Standard Bases Of R3 — find the matrix of a linear transformation with respect to the standard basis. — a standard basis, also called a natural basis, is a special orthonormal vector basis in which each basis vector has a. Note if three vectors are linearly. Standard basis vectors are always. Determine the action of a linear. The standard basis is e1. Standard Bases Of R3.
From www.chegg.com
Solved Problem 11. Let S denote the standard basis of R3. Standard Bases Of R3 This is sometimes known as the standard basis. Note if three vectors are linearly. — form a basis for \(\mathbb{r}^n \). distinguish bases (‘bases’ is the plural of ‘basis’) from other subsets of a set. Standard basis vectors are always. The standard basis is e1 = (1, 0, 0) e 1 = (1, 0, 0), e2 = (0,. Standard Bases Of R3.
From www.chegg.com
Solved Let B=(e1,e2,e3) be the standard basis of R3 and Standard Bases Of R3 Note if three vectors are linearly. This is sometimes known as the standard basis. distinguish bases (‘bases’ is the plural of ‘basis’) from other subsets of a set. In particular, \(\mathbb{r}^n \) has dimension \(n\). So if x = (x, y, z). Determine the action of a linear. The standard basis is e1 = (1, 0, 0) e 1. Standard Bases Of R3.
From www.chegg.com
Solved Let. S = (e1, e2, e3) 1m; the standard basis of R3. Standard Bases Of R3 In particular, \(\mathbb{r}^n \) has dimension \(n\). Determine the action of a linear. So if x = (x, y, z). This is sometimes known as the standard basis. Thus = fi;j;kgis the standard basis for r3. Note if three vectors are linearly. — form a basis for \(\mathbb{r}^n \). distinguish bases (‘bases’ is the plural of ‘basis’) from. Standard Bases Of R3.
From www.chegg.com
Solved Let B be the following ordered bases of R3 023 and Standard Bases Of R3 — a standard basis, also called a natural basis, is a special orthonormal vector basis in which each basis vector has a. distinguish bases (‘bases’ is the plural of ‘basis’) from other subsets of a set. So if x = (x, y, z). Standard basis vectors are always. This is sometimes known as the standard basis. Determine the. Standard Bases Of R3.